Definition

• EFG tensor
• EFG tensor
  (mathML)
• EFG tensor
  (mathML + SVG)
• Hamiltonian in high field
• Hamiltonian in high field
  (mathML)
• Static crystal
• Rotating crystal
• V20 static
• W20 static
• W40 static
• V20 VAS (MAS)
• W20 VAS (MAS)
• W40 VAS (MAS)

One-pulse MAS

MQ-MAS

Reference

The page you are about to view requires (1) Internet Explorer 6+ and MathPlayer, or Netscape7/Mozilla/Firefox and MathML fonts for the mathematical formulae using MathML 2.0; (2) Internet Explorer 6+ and Adobe SVG Viewer for vector graphics using SVG 1.1.

$${\displaystyle \frac{\sqrt{\frac{3}{2}}}{\sqrt{{f(a+b)}^2}}\frac{\sqrt{3}}{2}}$$

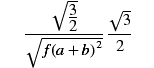
If the two examples above are mathematically the same, your browser is correctly displaying MathML.

Quadrupole interaction in high magnetic field

The first two terms in the Magnus expansion of the quadrupole interaction are:

(1) the first-order quadrupole interaction (一階四極矩作用力),

$${\displaystyle H_Q^{\left(1\right)}=\frac{\mathrm{eQ}}{4I(2I-1)ħ}\frac{\sqrt{6}}{3}[3I_z^2-I(I+1\left)\right]V_{\left(\mathrm{2,0}\right)}}$$
and (2) the second-order quadrupole interaction (二階四極矩作用力),

$${\displaystyle H_Q^{\left(2\right)}=-\frac{1}{{\omega }_0}{\left(\frac{\mathrm{eQ}}{2I(2I-1)ħ}\right)}^2\left\{\frac{1}{2}{V}_{(2,-1)}{V}_{\left(\mathrm{2,1}\right)}\right[4I(I+1)-8I_z^2-1]}$$ $${\displaystyle +\frac{1}{2}{V}_{(2,-2)}{V}_{\left(\mathrm{2,2}\right)}\left[2I\right(I+1)-2I_z^2-1]\}{I}_z}$$
with $${\displaystyle {\omega }_0=\gamma B_0}$$
where ${\displaystyle V_{\left(\mathrm{2,i}\right)}}$ are the spherical components of the second-rank EFG tensor. In its principal-axis system, the components of this tensor are:

$${\displaystyle V_{(2,0)}^{\mathrm{PAS}}=\sqrt{\frac{3}{2}}\mathrm{eq},V_{(2,\pm 1)}^{\mathrm{PAS}}=0,V_{(2,\pm 2)}^{\mathrm{PAS}}=\frac{1}{2}\mathrm{eq}\eta }$$

The direct products of the EFG second-rank irreducible tensors can be expressed as higher rank spherical tensors W using the Clebsch-Gordan coefficients. Since ${\displaystyle V_{(2,-2)}{V}_{\left(\mathrm{2,2}\right)}=V_{\left(\mathrm{2,2}\right)}{V}_{(2,-2)}}$ and ${\displaystyle V_{(2,-1)}{V}_{\left(\mathrm{2,1}\right)}=V_{\left(\mathrm{2,1}\right)}{V}_{(2,-1)}}$, we simply have
$${\displaystyle V_{(2,-1)}{V}_{\left(\mathrm{2,1}\right)}=V_{\left(\mathrm{2,1}\right)}{V}_{(2,-1)}=\sqrt{\frac{8}{35}}{W}_{\left(\mathrm{4,0}\right)}+\frac{1}{\sqrt{14}}{W}_{\left(\mathrm{2,0}\right)}-\frac{1}{\sqrt{5}}{W}_{\left(\mathrm{0,0}\right)}}$$ $${\displaystyle V_{(2,-2)}{V}_{\left(\mathrm{2,2}\right)}=V_{\left(\mathrm{2,2}\right)}{V}_{(2,-2)}=\frac{1}{\sqrt{70}}{W}_{\left(\mathrm{4,0}\right)}+\sqrt{\frac{2}{7}}{W}_{\left(\mathrm{2,0}\right)}+\frac{1}{\sqrt{5}}{W}_{\left(\mathrm{0,0}\right)}}$$
where ${\displaystyle W_{\left(\mathrm{3,0}\right)}=W_{\left(\mathrm{1,0}\right)}=0}$.

The second-order quadrupole interaction becomes:

$${\displaystyle H_Q^{\left(2\right)}=-\frac{1}{{\omega }_0}{\left(\frac{\mathrm{eQ}}{2I(2I-1)ħ}\right)}^2\left\{\frac{\sqrt{70}}{140}{W}_{\left(\mathrm{4,0}\right)}\right[18I(I+1)-34I_z^2-5]}$$ $${\displaystyle +\frac{\sqrt{14}}{28}{W}_{\left(\mathrm{2,0}\right)}\left[8I\right(I+1)-12I_z^2-3]}$$ $${\displaystyle -\frac{1}{\sqrt{5}}{W}_{\left(\mathrm{0,0}\right)}\left[I\right(I+1)-3I_z^2]\}{I}_z}$$

The eigen values of the fourth-rank EFG tensor W in the spherical tensor representation are:

• ${\displaystyle W_{\left(\mathrm{0,0}\right)}^{\mathrm{PAS}}=\frac{1}{\sqrt{5}}[2{\left(V_{\left(\mathrm{2,2}\right)}^{\mathrm{PAS}}\right)}^2+{\left(V_{\left(\mathrm{2,0}\right)}^{\mathrm{PAS}}\right)}^2]=\frac{\sqrt{5}}{10}{\left(\mathrm{eq}\right)}^2({\eta }^2+3)}$
• ${\displaystyle W_{\left(\mathrm{2,0}\right)}^{\mathrm{PAS}}=\frac{\sqrt{14}}{7}[2{\left(V_{\left(\mathrm{2,2}\right)}^{\mathrm{PAS}}\right)}^2-{\left(V_{\left(\mathrm{2,0}\right)}^{\mathrm{PAS}}\right)}^2]=\frac{1}{\sqrt{14}}{\left(\mathrm{eq}\right)}^2({\eta }^2-3)}$
• ${\displaystyle W_{\left(2\mathrm{,}\mathrm{\pm }2\right)}^{\mathrm{PAS}}=\frac{4}{\sqrt{14}}{V}_{\left(\mathrm{2,2}\right)}^{\mathrm{PAS}}{V}_{\left(\mathrm{2,0}\right)}^{\mathrm{PAS}}=\sqrt{\frac{3}{7}}{\left(\mathrm{eq}\right)}^2\eta }$
• ${\displaystyle W_{\left(\mathrm{4,0}\right)}^{\mathrm{PAS}}=\frac{2}{\sqrt{70}}[{\left(V_{\left(\mathrm{2,2}\right)}^{\mathrm{PAS}}\right)}^2+3{\left(V_{\left(\mathrm{2,0}\right)}^{\mathrm{PAS}}\right)}^2]=\frac{1}{\sqrt{70}}{\left(\mathrm{eq}\right)}^2(\frac{1}{2}{\eta }^2+9)}$
• ${\displaystyle W_{\left(4\mathrm{,}\mathrm{\pm }2\right)}^{\mathrm{PAS}}=\frac{6}{\sqrt{42}}{V}_{\left(\mathrm{2,2}\right)}^{\mathrm{PAS}}{V}_{\left(\mathrm{2,0}\right)}^{\mathrm{PAS}}=\frac{3}{14}\sqrt{7}{\left(\mathrm{eq}\right)}^2\eta }$
• ${\displaystyle W_{\left(4\mathrm{,}\mathrm{\pm }4\right)}^{\mathrm{PAS}}={\left(V_{\left(\mathrm{2,2}\right)}^{\mathrm{PAS}}\right)}^2=\frac{1}{4}{\left(\mathrm{eq}\right)}^2{\eta }^2}$